2D Graphics Generated by the Wolfram Language

This page contains a number of examples of 2D graphics created in the Wolfram Language. Click on the thumbnail to view a full-sized version.

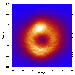	Doppler Tomography Doppler tomography of a cataclysmic variable star system. Doppler tomography is a method of obtaining a map of gas distribution from spectroscopy when the system itself cannot be resolved optically. Created by Jeff Bryant (jeffb@wolfram.com)
	3 Body Problem A solution to the restricted 3-body problem. In this case, it is applied to a cataclysmic variable star system and shows the path of a gas particle being transferred from a lower mass secondary star to the more massive primary star. Created by Jeff Bryant (jeffb@wolfram.com)
	Riemann Zeta ContourPlot of the Riemann Zeta function in the complex plane. Created by Jeff Bryant (jeffb@wolfram.com)
	Spectral Portraits Spectral portrait of a 655x655 sparse matrix. The spectral portrait is a graphical tool that provides the user with information about the spectral sensitivity of the spectrum of a matrix. Created by Jeff Bryant (jeffb@wolfram.com)
	Adaptive Sampling Adaptive sampling of 2D graphics. Mathematica adaptively determines the sampling of the points used to construct curves in 2D. More points are used only where necessary. Created by Jeff Bryant (jeffb@wolfram.com)
	Sparse Matrices 76480 node sparse matrix connectivity using GraphPlot. Created by Jeff Bryant (jeffb@wolfram.com)